Mathematics Awareness Month 2014: Mathematics, Magic, and Mystery

Navigate the Calendar

Rope Tricks

Here is mathematics professor and knot theorist Louis H. Kauffman to demonstrate a few rope tricks:

And here are some explanations:

Taking it Further

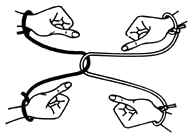
For your first challenge problem, here’s an old party trick for two people that is fun to do and even more fun to watch. (For the sake of discussion, suppose the two people are male and female.) First, tie the man’s wrists together manacle-style with a rope. Tie the wrists loosely “for his comfort,” but tell him he can’t take the rope off his wrists. Tie the woman’s wrists together in the same manner, linking her rope with his, as shown below. The challenge is for the two people to unlink themselves without untying the ropes.

Dr. Kauffman states several times in the videos above that a knot can always be undone if we simply “slide it off the end of the rope.” In the mathematical theory of knots, this action is explicitly forbidden—by joining the two ends of the rope after the knot is tied. That is, a mathematical knot is a circle embedded in three-dimensional space. The theory of knots is a well-developed branch of mathematics that has seen significant development over the past century.

One of the central questions in the theory of knots is their classification: How many distinct knots are there? The task of assembling a definitive knot atlas has been in progress for over a century. The main difficulty lies in discerning whether any two given knots are in fact different. Can one be manipulated into the other?

For your second challenge, inspect the two knots below. They were cataloged as distinct knots at the turn of the last century, and the error persisted for decades: Only in 1973 was it discovered that they are in fact the same knot! Can you manipulate one into the other to show they are in fact the same? Get a long piece of string and lay it out as shown in the left knot before fastening the ends together. With the ends securely fastened, see if you can gently manipulate it into the knot on the right. The discovery that the two knots are the same was made by Kenneth Perko, and the pair have come to be known as the Perko pair.

There are literally thousands of rope tricks, and many offer compelling insight into mathematical ideas. For your final challenge, take a look at the following short video, and see if you can determine why removing a single “nail” or “finger” leads to the unraveling of the rope. (Note: The word “tree” that appears in the first few seconds of the video should be “three.”) A written account of the same ideas can be found in the blog post Hanging Pictures and Homology Groups by Sachi Hashimoto.

The Underlying Mathematics

Rope and knot tricks have been a staple of magicians for centuries. Most famously controversial was the Indian Rope Trick, dating from nineteenth century or earlier. In its most basic form, this trick involved a coiled rope left in a basket that would levitate, with no support. A boy would then climb up the rope into thin air. However, despite being engrained in popular western culture through word of mouth, written materials, and movies, no credible evidence exists that the trick was ever actually performed!

A key development in knot theory was the discovery of certain (easily computable) polynomials that are known to be knot invariants. The Alexander polynomial of a knot, sometimes called the Alexander-Conway polynomial, was discovered by James W. Alexander in 1923. In 1969 John H. Conway invented a new notation for knots and used it to classify, by hand, a large collection of knots. Later it was discovered that Conway’s approach did essentially what Alexander’s did. But only in 1984, with Vaughn Jones’ discovery of a new polynomial (now called the Jones Polynomial), did the true significance of these polynomial invariants become clear. They are now standard tools used in the analysis of knots.

Louis Kauffman, shown in the videos above, discovered a generalization of the Jones polynomial, known as the Kauffman polynomial, and a model for the Jones polynomial, the bracket polynomial, that is notable for its simplicity, its relationship with statistical mechanics, and its relationship with further generalizations such as Khovanov homology. He is the author of a number of books on knots, including Formal Knot Theory (Princeton University Press, 1983; and Dover, 2006), On Knots (Princeton University Press, 1987) and Knots and Physics (World Scientific, 2001).

A slew of rope tricks, including the first challenge problem and several of Dr. Kauffman’s tricks as presented in the videos, are described in Martin Gardner’s Mathematics, Magic and Mystery (Dover, 1956). See chapter 5, “Topological Tomfoolery.”

Graham and Diaconis discuss the “inside-outside” magic of Stewart Judah in chapter 10 of Magical Mathematics: The Mathematical Ideas That Animate Great Magic Tricks (Princeton University Press, 2012). These effects make use of a closed loop of string or thin chain laid on a tabletop. The subject is asked to place a finger on the inside of the closed loop, which can be devilishly difficult if the chain is laid out in a certain way. The chapter also includes a delightful loop trick attributed to Dai Vernon.

A formal but elementary introduction to knot theory can be found in Colin Adams’ The Knot Book: An Elementary Introduction to the Mathematical Theory of Knots (American Mathematical Society, 2004). Adams also penned the comic book Why Knot?: An Introduction to the Mathematical Theory of Knots (Key College Publishing, 2004), which makes the subject of knot theory accessible to a younger audience.

The elegant solution to the “handcuff” challenge can be found here. The solution to the “Perko pair” challenge problem can be found here. Kenneth Perko’s published description is “On the classification of knots,” Proc. Amer. Math. Soc. 45 (1974), 262–266.