Mathematics Awareness Month 2014: Mathematics, Magic, and Mystery

Navigate the Calendar

The Gilbreath Principle

Here is Eve Torrence performing a version of the “Magnetic Colors” card trick designed by Norman Gilbreath:

Here is renowned mathematician Ron Graham demonstrating a seemingly different card trick. Notice that in both cases, the subject is permitted to shuffle the cards before the trick is performed:

Taking it Further

The two tricks performed above may seem completely unrelated, but in both cases the subject is permitted to shuffle the cards once. In both cases, the deck also starts in some predetermined order. A single shuffle disturbs that order, but does not obliterate it completely.

In the second video, the subject shuffles the cards by first dealing off about half of the deck, face down, to form a pile, and then riffling that pile together with the remainder of the deck. Such a procedure is known as a Gilbreath shuffle.

A quick and dirty (but not rigorous) explanation of the Gilbreath principle appeared in Jim Morton’s Pop Void blog entry “Understanding the Gilbreath Principle” in March, 2014, where the beauty and simplicity of the principle is laid bare. After giving that a read, see if you can answer the following challenge questions:

1. In the first video, the deck is prearranged so the cards alternate red, black, red, black,... all the way down. No matter how the subject cuts and riffle-shuffles the deck (perfectly or imperfectly), show that at most two cards of the same color can appear in sequence.
2. In that same trick, show that after the shuffle, if a pair of red cards are adjacent, then then the next pair of adjacent same-color cards (if there is one) must be black. Note that this implies there will be an even number of alternating cards between the two same-color pairs.
3. Show that cutting the deck in a way that spits one same-color pair will set up the deck so that when the cards are dealt out in pairs, every pair contains one red and one black card.
4. In the first video, a Gilbreath shuffle is not performed: The deck is simply cut, and the two halves are riffled together. The trick performed in the first video could be simplified if the subject instead performed a Gilbreath shuffle; after the shuffle it would no longer be necessary to cut the deck between two cards of the same color. Explain.

The Underlying Mathematics

An excellent reference for the Gilbreth Principle in its general form, and for a host of other mathematical magic effects, is the award winning Magical Mathematics: The Mathematical Ideas That Animate Great Magic Tricks, by Persi Diaconis and Ron Graham (Princeton University Press, 2012). Princeton University Press has graciously released chapter 5, “From the Gilbreath Principle to the Madelbrot Set,” online in honor of Mathematics Awareness Month.

In this chapter, Diaconis and Graham explain a subtle but profound connection between Gilbreath permutations and the Mandelbrot set, one of the most important constructs in modern mathematics. It is likely that there are new discoveries to be made that exploit this connection.

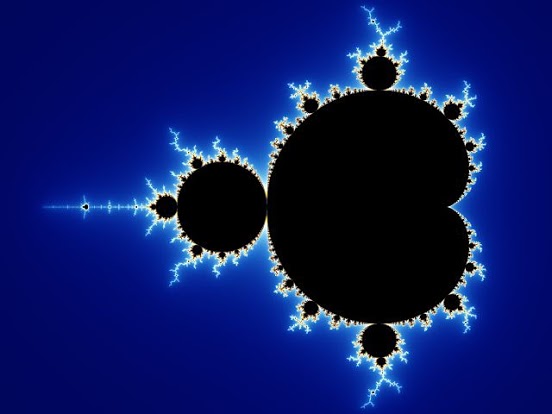
Martin Gardner wrote about the Gilbreath principle in his book Mathematical Magic Show (Mathematical Association of America, 1989). See chapter 7: “Playing Cards.” The book is also included on the CD compilation Martin Gardner’s Mathematical Games: The Entire Collection of His Scientific American Columns on one CD (MAA, 2008).

Several card tricks based on the Gilbreath principle are outlined in Colm Mulcahy’s Card Colm entry from August, 2005: “The First Norman Invasion,” and from August, 2006: “The Second Norman Invasion.”