Mathematics Awareness Month 2014: Mathematics, Magic, and Mystery
Navigate the Calendar
What’s the Point of a Vanishing Point?
Dick Termes draws, not on a flat canvas, but on a sphere. If you look at the Common Ground spinning sphere (in the first video below), you’ll see it turning from left to right. After about 30 seconds, the camera zooms in. If you get your face in close and use your hands to block out everything but the sphere, it will put you “in the space.” You should feel like you’re in the restaurant, watching the room spin backwards around you!
A similar shift happens about one minute into this second video of the sphere, Reflecting Back. Drawing our three-dimensional world onto a two-dimensional canvas requires some mathematical sleight-of-hand. And the inverse problem is intriguing, too! Figuring out how to look at a picture so that it “pops” back into 3D is a great exercise in geometry.
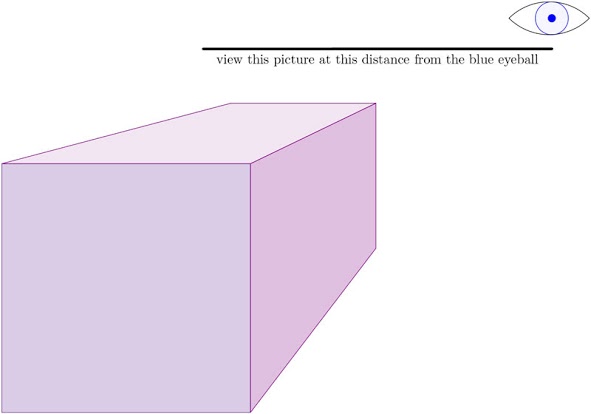
A more basic illusion that depends on your distance to the picture is shown below. From far away, this drawing looks like an image of a distorted, possibly rectangular box. But if you close one eye and move your face so that your open eye is directly in front of the picture of the eye, and so that it is as far from the picture as the distance indicated, then the picture will seem to be that of a perfect cube. The place where you stand influences the picture that you see!
Taking it Further
You can create your own perspective illusions by “forcing” perspective, as in this photo below.

Thinking again about the ideal viewing location for the box image in the previous section, here’s a challenge: Given a drawing or painting made in one point perspective (like the examples below), determine how far your eye should be from the image so that the illusion of perspective will be optimal. Or even more to the point: Describe a procedure for making this determination in a systematic way. All the clues you need are right there in the images! Hint: You need a perfect square in the “floor” of the image, two of whose sides extend to the vanishing point. Such a square has been provided in each of the images below. For another hint, take a peek at step 23(c) in Rick Faloon’s article “Teaching Leonardo: An integrated approach – Tiling a floor using Geometer’s Sketchpad.”


The Underlying Mathematics
How does Termes’ illusion work? You can see in Cubical Universe (video below) that Termes uses what he calls “6-point perspective.” He imagines he is sitting in the exact center of the sphere looking out. As a consequence, vertical lines all appear to converge at the north and south poles; east-west lines converge at a pair of opposite points on the equator, and north-south lines converge at another pair of points on the equator.
When you are far from the sphere, you are far away from Termes’ imagined viewpoint, so you see the sphere. As you move close to the sphere, you are moving closer to his viewpoint, so you are seeing what he saw: you see the room.
Similarly, if you look at the box from the same distance as the artist who drew the box, it will look “right” in a way that it doesn’t when you’re far from the picture.
The mathematics of vanishing points and viewpoints helps artists draw realistic looking pictures. It is very closely related to the theoretical subject of projective geometry. This magical math also allows the rest of us to see the world—through the art—in a whole new way.
An excellent reference for the mathematics of perspective is Viewpoints: Mathematical Perspective and Fractal Geometry in Art, by Marc Frantz and Annalisa Crannell (Princeton University Press, 2011). Chapter 3 explains in detail how to determine the correct viewing distance to a painting; it is something that comes in handy when you visit a museum!
Annalisa Crannell gives her (ahem) perspective on a myriad of visual topics on her blog Perspective Geometry.