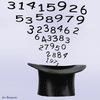Mathematics Awareness Month 2014: Mathematics, Magic, and Mystery
Navigate the Calendar
Ambigrams
What reads the same right side up and upside down? What combines mathematics, art, and language? An ambigram! These three videos show you examples of ambigrams, and explain how to create them.
Taking it Further
Once you understand the basic idea of ambigrams, you are ready for other challenges involving letters and symmetry.
- CLASSIFY the 26 capital letters of the alphabet by symmetry. Which letters are symmetrical? Which have no symmetry? How many different kids of symmetry are there? Which letters have more than one kind of symmetry? Here is the full activity.
- HALF WORDS. An intriguing and challenging warmup exercise that is an excellent way to get kids excited about symmetry. Put half words together to make whole words. Handout. Teacher notes.
- MIRROR DRAWING. An exciting two-person drawing game that makes a good introduction to concepts of symmetry. Handout. Teacher notes.
- INVERSIONS NAMES. An intriguing design with 26 first names, one for each letter of the alphabet, each an inversion. A good way to introduce rotational and reflective symmetry, as well as different lettering styles. Handout. Teacher notes.
- THINKING UPSIDE DOWN. Here’s an eight-page booklet that includes all of the activities above, and many more.
- CREATE your own ambigrams on names of mathematical ideas, or names of mathematicians. Here are some mathematically-themed ambigrams created by mathematician/artist/juggler Burkard Polster.
- READ the story behind the MATH MAGIC ambigram that appears on the Math Awareness Month poster.
- VISIT a gallery of my ambigrams, many of which are animated.
- LEARN the secrets of creating ambigrams from typographic designer and prolific ambigram creator John Moore of Venezuela. And here's my take on how to create your own ambigrams.
The Underlying Mathematics
Ambigrams are based on the mathematics of symmetry. The four basic geometric transformations are translation (sliding a shape in the plane without turning it), rotation (turning a shape about a center-point), reflection (reflecting a design about a mirror), and scaling (changing the size of a shape). Most ambigrams are based on rotation or reflection; a few are based on scaling or translation.
For a given plane figure, such as a square, rectangle, equilateral triangle, or something more complex, the set of symmetries for that figure form a natural algebraic system called a group. If the geometric figure is an ambigram, it will likely have either 180-degree rotational symmetry, or reflective symmetry through a horizontal or vertical line.
To learn more about how geometric transformation appear in mathematics, art, music and animation, see Scott Kim speaking on Symmetry, Art and Illusion, part of the Math Encounters series at the Museum of Mathematics.