Mathematics Awareness Month 2014: Mathematics, Magic, and Mystery
Navigate the Calendar
Fold and One Cut
Can you cut out a perfect five-pointed star from a sheet of paper with a single straight cut? It is claimed (probably falsely) that Betsy Ross knew how to do it, and Harry Houdini wrote about it in his 1922 book Paper Magic. It’s easy:
So what other shapes can be made from a sheet of paper by folding it and then making a single straight cut? Or maybe a better question is: What shapes cannot be made this way?
Taking it Further
Here are some simple challenges taken from The Art of Mathematics to get you thinking about how to design these patterns.
- Draw an equilateral triangle. Can you fold the paper and then use one cut to cut it out?
- Next try to do the same thing with a square.
- The examples above and in the videos are shapes with rotational symmetry. Is it necessary for a shape to have rotational symmetry to be possible to make it with one cut?
- Draw an irregular polygon with four sides. Can you fold this shape and cut it out with one cut?
- How about an irregular polygon with five sides?
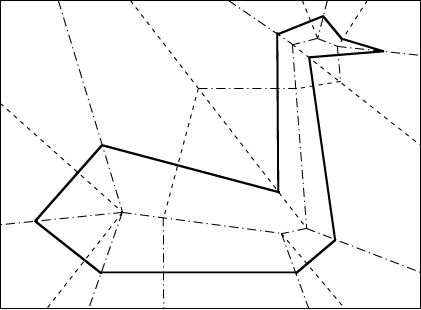
The pattern for the swan shown below and several other designs by Erik Demaine, along with directions for folding them, can be found here. These patterns include the fold lines. The dash and dot lines are mountain folds, and the dash lines are valley folds. The swan can be cut out with a single straight cut after the paper is folded! Can you do it?
The Underlying Mathematics
Martin Gardner wrote about the fold-and-cut problem in his famous “Mathematical Games” column in Scientific American. His writing on paper cutting can be found in chapter 5 of Sphere Packing, Lewis Carroll, and Reversi: Martin Gardner’s New Mathematical Diversions. He leaves it as an open problem to determine which polygons can be obtained via fold-and-one-cut.
In 1998, Erik Demaine, Martin Demaine, and Anna Lubiw solved this decades old problem when they proved the fold-and-cut theorem. The theorem asserts that any shape with straight sides can be cut from a single (idealized) sheet of paper by folding it flat and making a single straight complete cut.
Joseph O’Rourke briefly describes two fold-and-one-cut methods in his Computational Geometry Column 36, in The International Journal of Computational Geometry and Applications (Vol. 9 No. 6, 1999, pp 615–618).
You can watch Professor Demaine give a lecture on fold-and-one-cut, from his course “Geometric Folding Algorithms: Linkages, Origami, Polyhedra” (Fall 2010).