Current MAM Home Page || Previous MAWs/MAMs || Current Activities

Many geophysical flows of interest are effectively two-dimensional due to the Earth's rotation and the stable stratification of the ocean. The geometrical approach to the fluid transport in such flows is based on identifying regions of the flow with distinct motion type (e.g. recirculating in a vortex) and following their evolution. In case of the flow with periodic time dependence, the key objects, separating the phase space into regions with different types of dynamics, are the stable and unstable invariant manifolds (IM) of the saddle-type trajectories.

Recently this approach was extended to the general 2D flows, including numerically generated velocity fields, defined only on a finite time interval. We apply the dynamical systems methodology to investigate the Lagrangian dynamics of the Loop Current and the adjacent mesoscale rings in the eastern part of the Gulf of Mexico. Observational data (altimetry, drifters) and a model velocity field (University of Colorado real-time nowcast/forecast version of the Princeton Ocean Model) are used in the study. A Lagrangian approach provides detailed information about ring interaction and reveals features of the ring dynamics that are not apparent in the Eulerian picture.

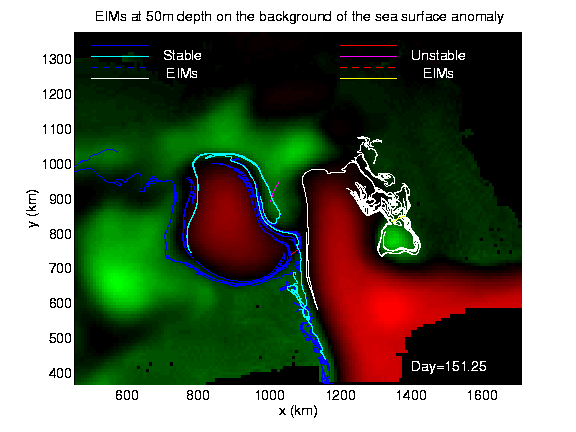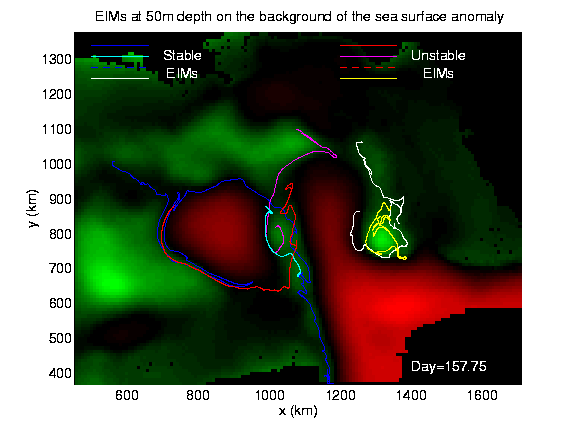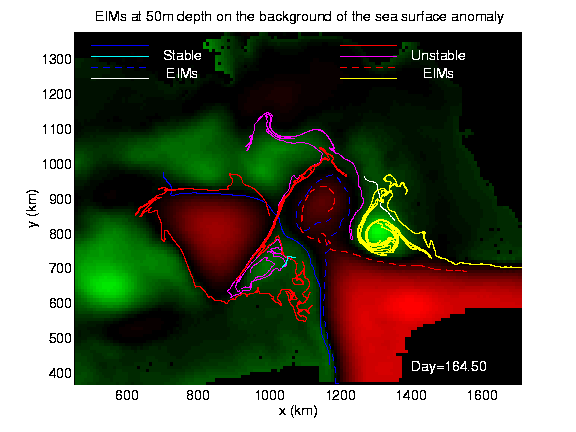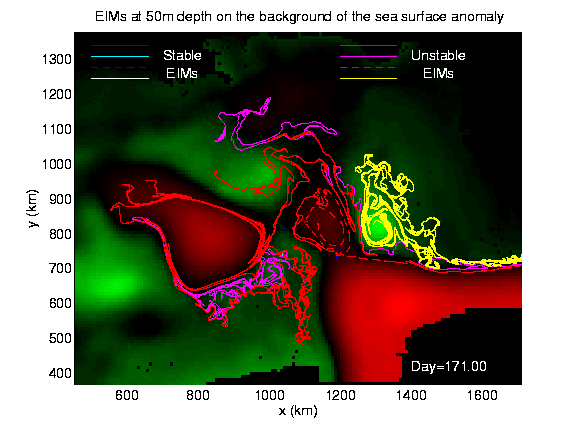Watch as a QuickTime movie | Download the AVI movie

During the 20-day observation period, a new ring was formed in the meander of the Loop Current and a large anticyclonic ring to the west was cleaved by a cyclonic eddy. The coherent structures were identified in the Lagrangian framework by means of Effective Invariant Manifolds -- material lines that play the analogous role as IMs do in periodic case. EIMs divide the flow into the regions with distinguished dynamic fate, acting as boundaries between coherent structures.

This work was done by a collaboration of researchers from Brown University (L. Kuznetsov and C.K.R.T. Jones), University of Delaware (M. Toner and A.D. Kirwan) and University of Colorado (L. Kantha and J. Choi).